Hydrogen Bromide Molecular
Orbital Calculations
The optimized geometry of hydrogen bromide (HBr) was based on the Double Zeta Valence (DZV) calculation. The bond length was determined for that geometry.
|
|
The electrostatic potential of HBr was mapped on the molecule. Red denotes high electron density, and blue denotes low electron density.
|
|
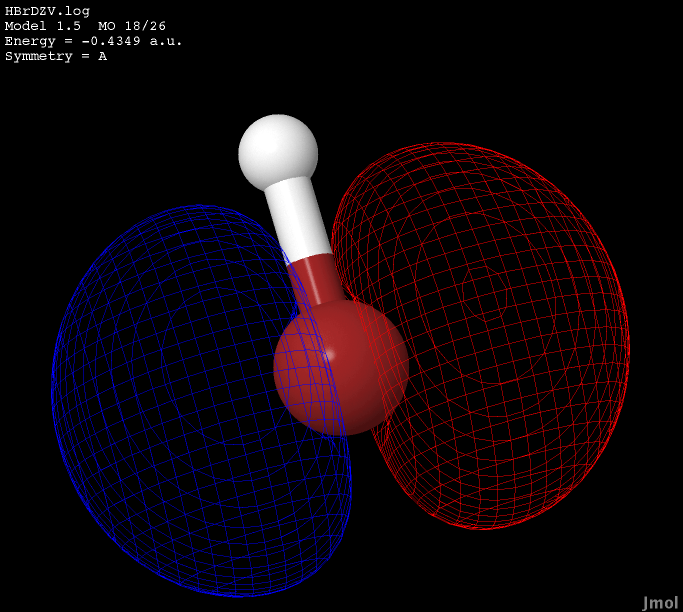
The Highest Occupied Molecular Orbital (HOMO) for HBr was calculated using DZV.
|
|
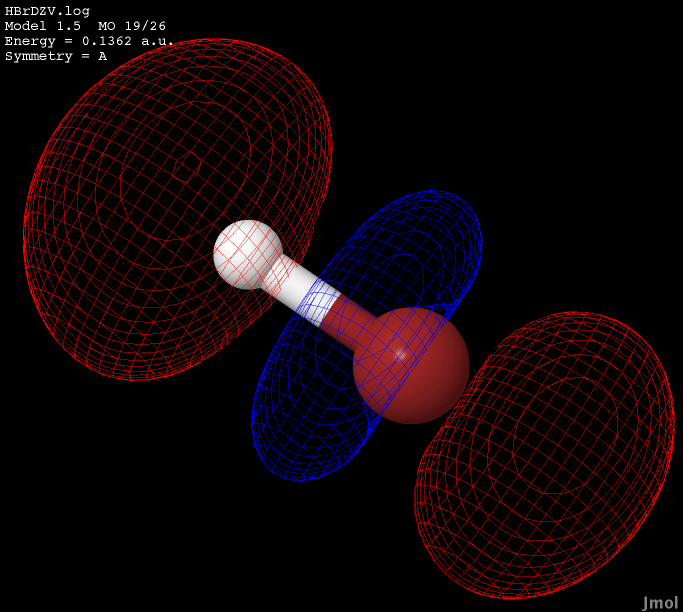
The Lowest Unoccupied Molecular Orbital (LUMO) for HBr was determined with the DZV level of theory.
|
|
The partial charges on hydrogen and bromine were determined based on the DZV model.
|
|
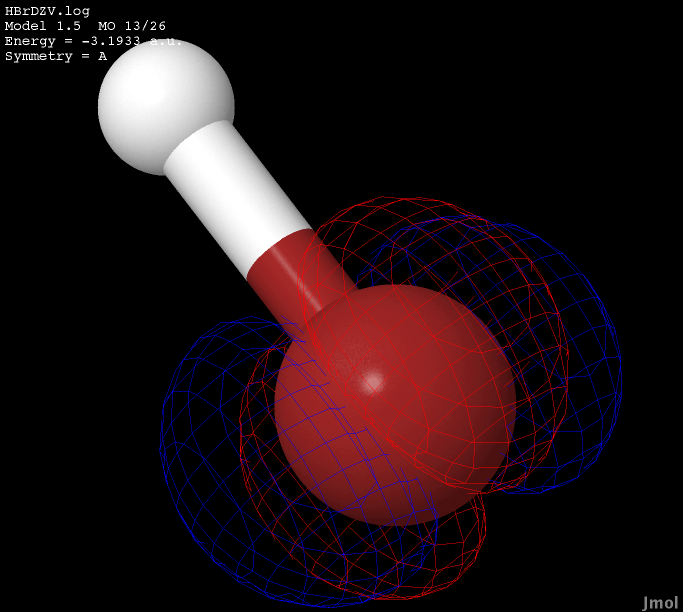
Table 1: HBr Molecular Orbitals and their corresponding energies and occupation numbers.
| Occupation Number |
Energy (Hartrees) |
Bonding |
Molecular Orbital |
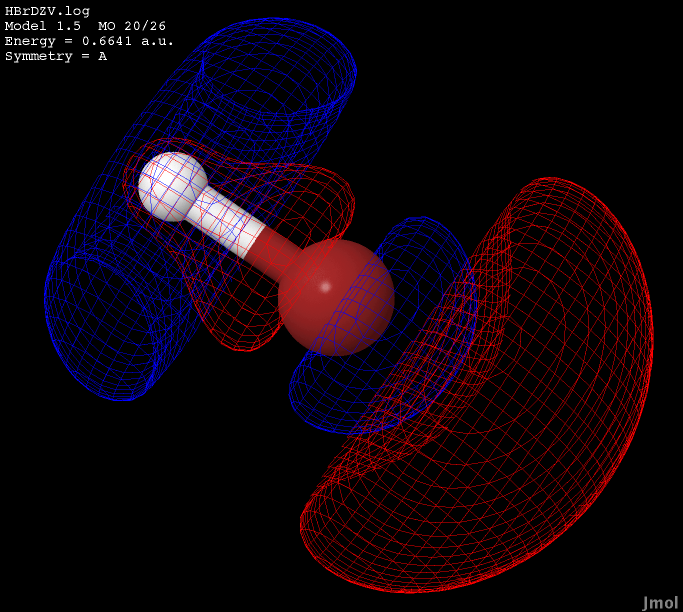
| 0 |
0.6641 |
nonbonding |
 |
| 0 |
0.1362 |
antibonding |
 |
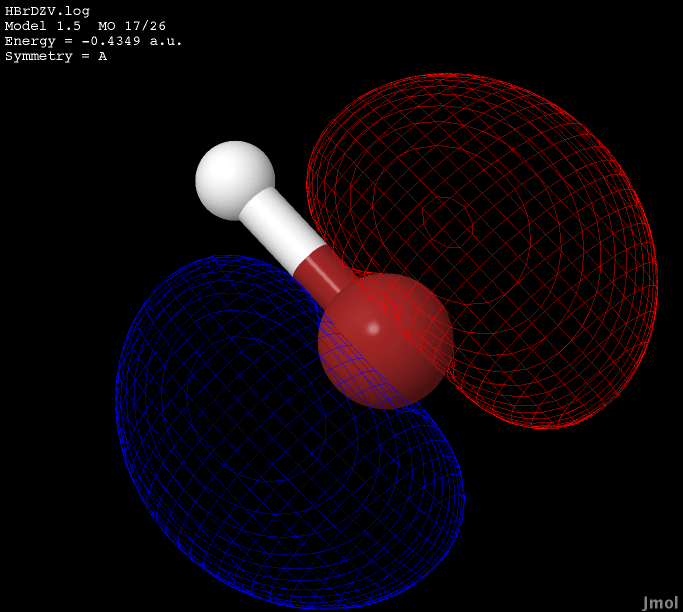
| 2 |
-0.4349 |
nonbonding |
 |
| 2 |
-0.4349 |
nonbonding |
 |
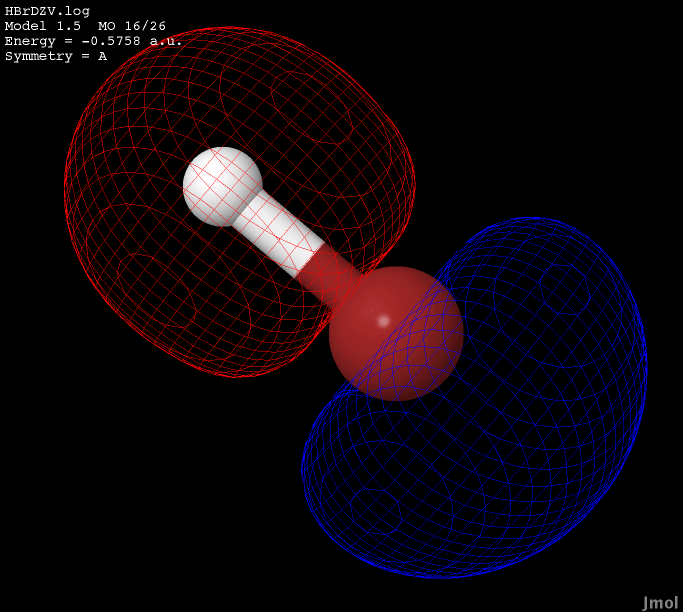
| 2 |
-0.5758 |
nonbonding |
 |
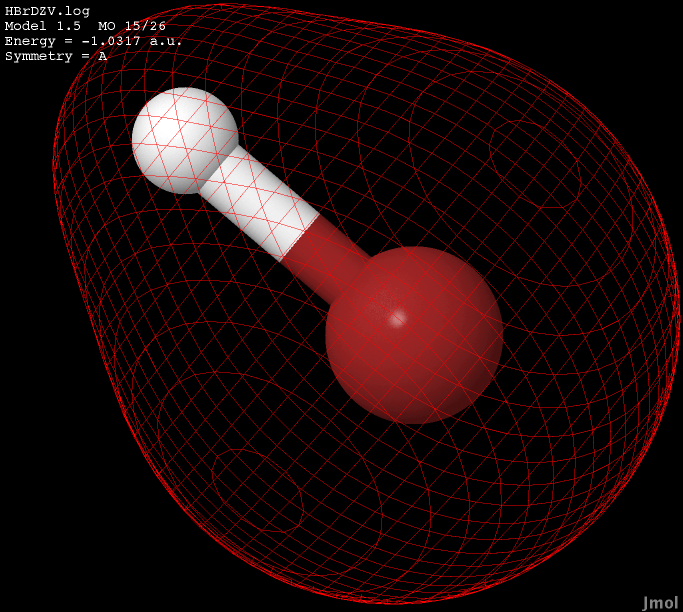
| 2 |
-1.0317 |
bonding |
 |
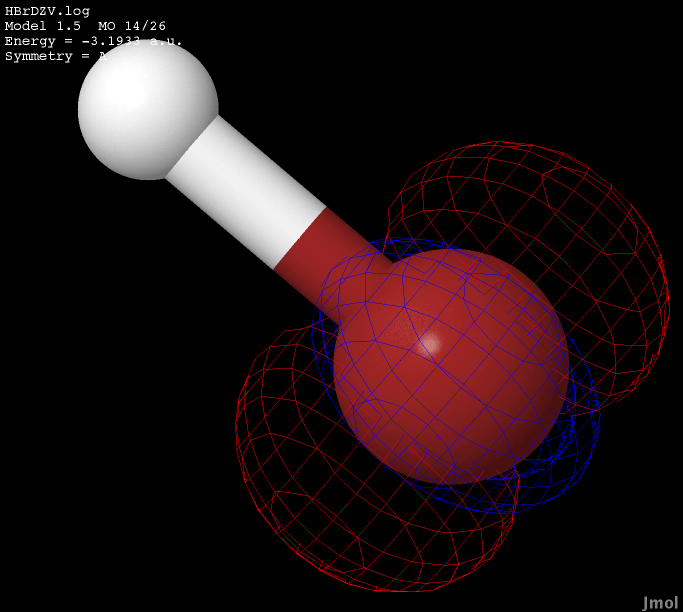
| 2 |
-3.1933 |
nonbonding |
 |
| 2 |
-3.1933 |
nonbonding |
 |
| 2 |
-3.2094 |
nonbonding |
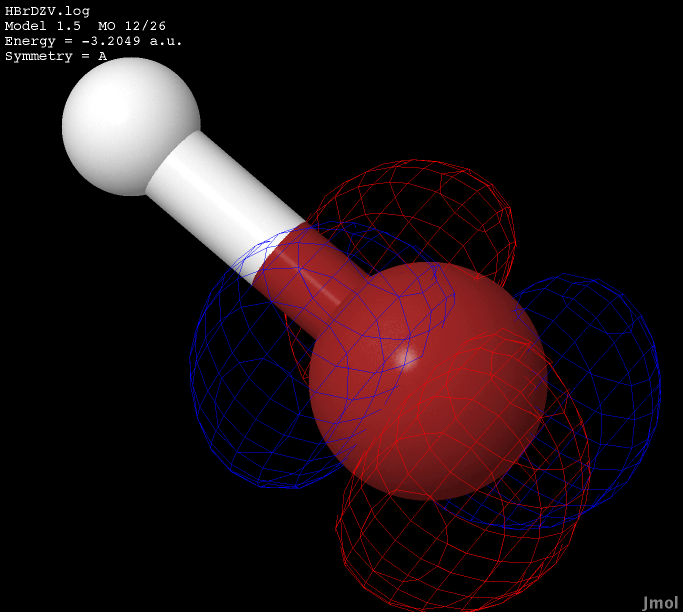 |
| 2 |
-3.2094 |
nonbonding |
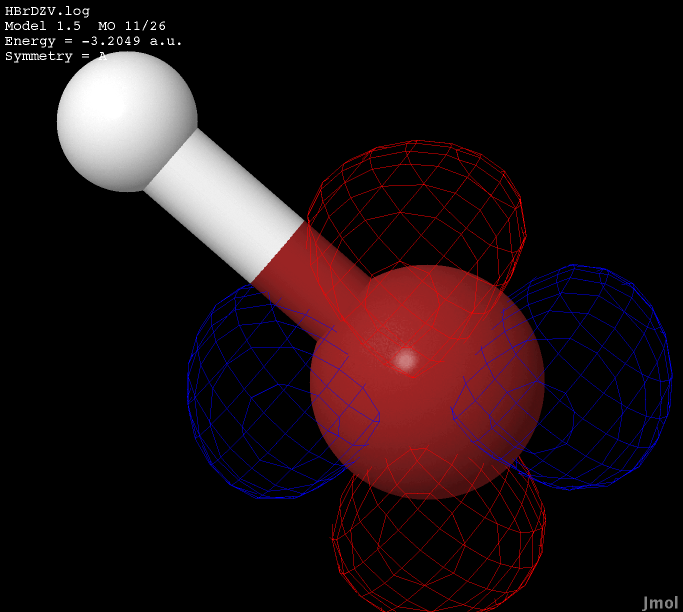 |
| 2 |
-3.2094 |
nonbonding |
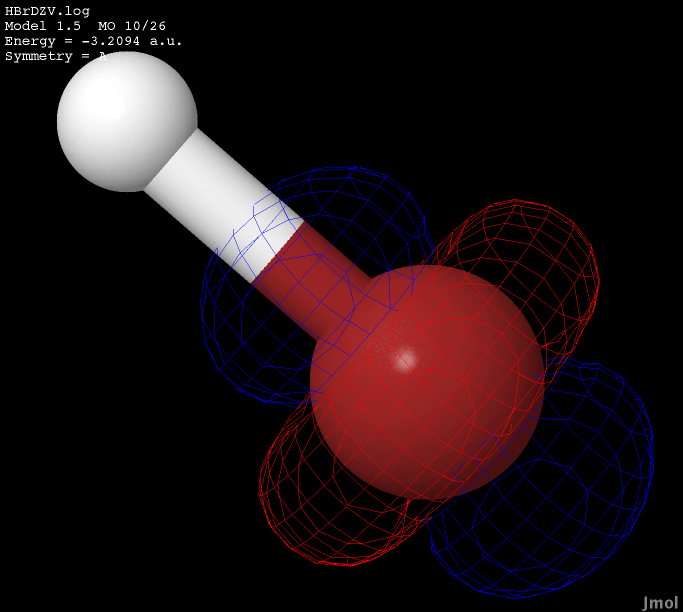 |
| 2 |
-7.4577 |
nonbonding |
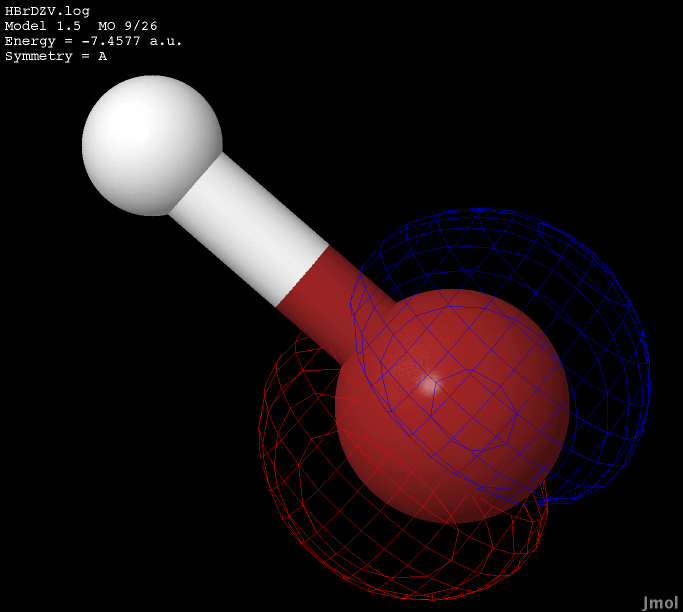 |
| 2 |
-7.4577 |
nonbonding |
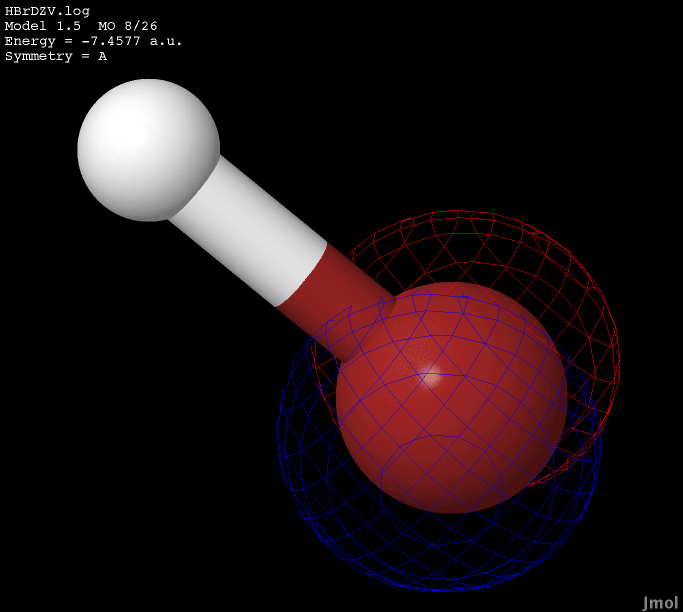 |
| 2 |
-7.4684 |
nonbonding |
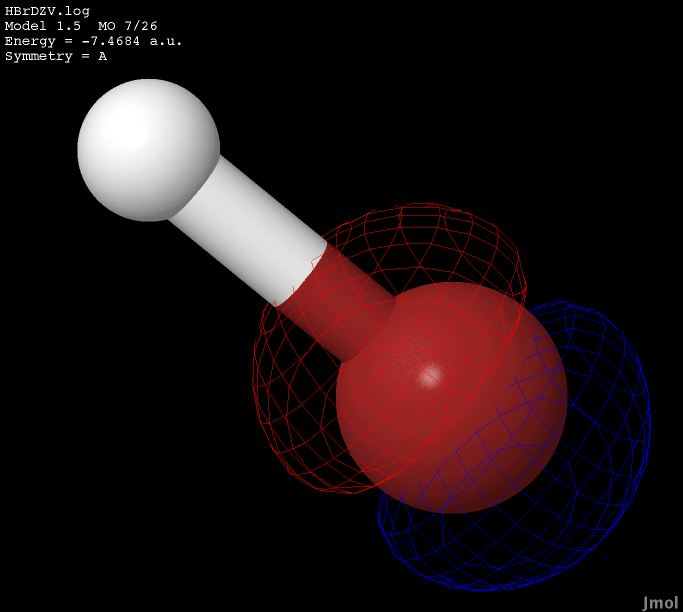 |
| 2 |
-9.7026 |
nonbonding |
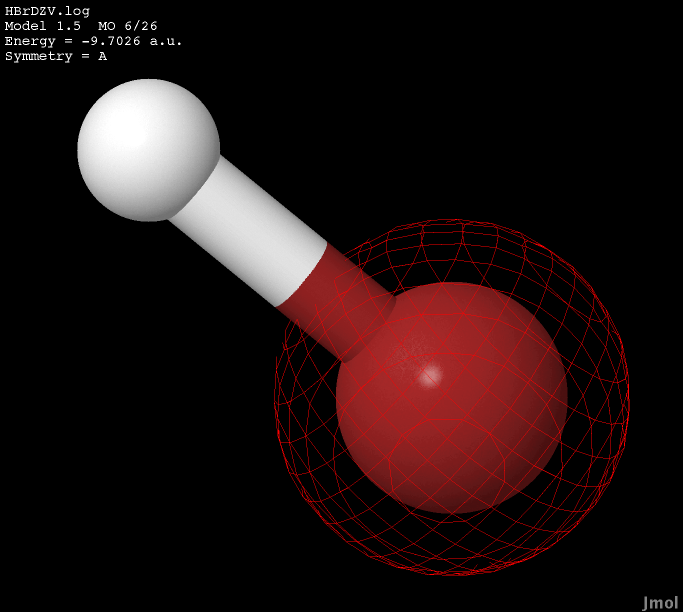 |
Figure 1: The molecular orbital diagram of
HBr. The * signifies antibonding orbitals.
Table 2: The dipole moments calculated at different levels of theory and with different diffuse functions.
| (D,F,L) |
3-21G Dipole Moment
(D) |
(D,F,L) |
6-31G Dipole Moment
(D) |
(D,F,L) |
DZV Dipole Moment
(D) |
| (1,0,1) |
1.050075 |
(1,0,1) |
1.146264 |
(1,0,1) |
1.097927 |
| (2,0,2) |
0.855275 |
(2,0,2) |
0.978206 |
(2,0,2) |
0.950998 |
| (3,0,3) |
0.893857 |
(3,0,3) |
0.951680 |
(3,0,3) |
0.961316 |
D=# D heavy atom polarization functions
F=# F heavy atom polarization funtions
L=# Light atom polarization functions
Table 3: The most accurate dipole moment value with the corresponding theoretical value.1
| Calculated (3-21G) |
0.893857D |
| Theoretical |
0.827D
|
The best dipole moment was determined to be the value calculated based on the 3-21G optimization. For this particular molecule, the larger basis sets resulted in dipole moment values that were higher than expected. The smaller basis sets yielded more reasonable dipole moment values because they were smaller.
The calculated vibrational frequency of HBr was found to be 2635.8cm-1.
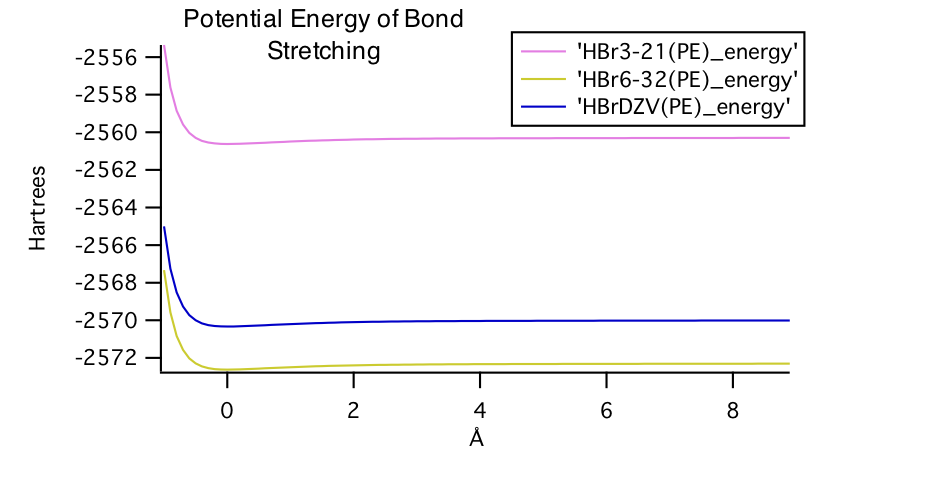
Figure 2: The potential energy of bond stretching for HBr. The three different levels of theory are all plotted on this graph.
In Figure 1, 3-21G had the highest energy basis set, and 6-31G had the lowest energy basis set. The larger basis sets were considerably lower energy than the smallest basis set, although the largest basis set was not the lowest energy.
References:
1. Lide, D. R. CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, 1992.
Page
skeleton and JavaScript generated by export to web function
using Jmol 12.2.34
2012-08-09 20:37 on Mar 3, 2013.